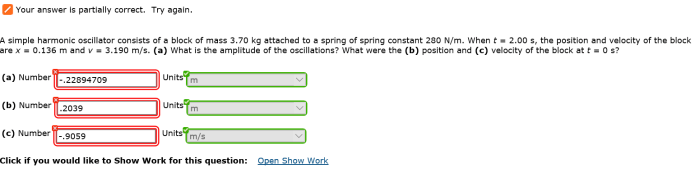
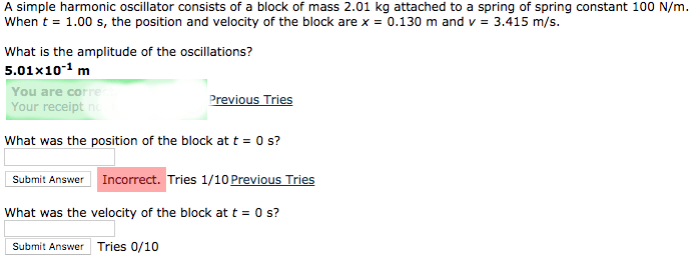A Simple Harmonic Oscillator Consists Of A Block Of Mass

A simple harmonic oscillator consists of a block of mass – A simple harmonic oscillator, consisting of a block of mass, provides a fundamental understanding of oscillatory systems. Its components and properties, governed by the equation of motion, play a crucial role in its behavior and energy considerations. This exploration delves into the applications of simple harmonic oscillators, highlighting the influence of the block of mass on their functionality.

The concept of a block of mass in a simple harmonic oscillator forms the cornerstone of this investigation, as its properties dictate the oscillator’s dynamics and energy relationships.

Introduction to Simple Harmonic Oscillator: A Simple Harmonic Oscillator Consists Of A Block Of Mass

A simple harmonic oscillator (SHO) is a system that undergoes periodic motion around an equilibrium point with a restoring force proportional to its displacement from the equilibrium point. It is characterized by a block of mass attached to a spring or other elastic medium, which allows it to oscillate back and forth.

The block of mass is an essential component of a SHO, as it provides the inertia necessary for the system to oscillate. Without the block of mass, the system would not have the ability to store and release energy, resulting in a lack of oscillation.

Components and Properties, A simple harmonic oscillator consists of a block of mass

Spring:A spring is an elastic device that stores energy when stretched or compressed. In a SHO, the spring provides the restoring force that pulls the block of mass back to its equilibrium point.
Block of Mass:The block of mass is the object that oscillates in a SHO. Its mass determines the inertia of the system and affects the frequency of oscillation.
Equilibrium Point:The equilibrium point is the position where the block of mass is at rest. The restoring force is zero at this point.
Amplitude:The amplitude is the maximum displacement of the block of mass from its equilibrium point.
Frequency:The frequency is the number of oscillations per unit time. It is determined by the mass of the block and the stiffness of the spring.

Equation of Motion

The equation of motion for a SHO is given by:

$$F =

kx$$

where:

$F$ is the restoring force
$k$ is the spring constant
$x$ is the displacement from the equilibrium point

This equation shows that the restoring force is directly proportional to the displacement, which means that the greater the displacement, the greater the force pulling the block of mass back to equilibrium.

Energy Considerations

The total energy of a SHO is the sum of its kinetic and potential energies. The kinetic energy is the energy of motion, while the potential energy is the energy stored in the spring. The total energy of a SHO is constant, and it is given by:

$$E = \frac12kA^2$$

where:

$E$ is the total energy
$k$ is the spring constant
$A$ is the amplitude

This equation shows that the total energy of a SHO depends on the spring constant and the amplitude of the oscillation.

Applications

SHOs have numerous applications in various fields, including:

Pendulums:Pendulums are a type of SHO that is used to measure time.
Springs:Springs are used in many devices, such as shock absorbers and musical instruments.
Resonance:Resonance is a phenomenon that occurs when a system is driven at its natural frequency. It can be used to amplify vibrations or to create standing waves.

The block of mass plays a crucial role in the applications of SHOs. It determines the frequency of oscillation, the total energy, and the amplitude of the motion.

jordin

A Simple Harmonic Oscillator Consists Of A Block Of Mass

Introduction to Simple Harmonic Oscillator: A Simple Harmonic Oscillator Consists Of A Block Of Mass